Mi SciELO
Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO -
 Accesos
Accesos
Links relacionados
-
 Citado por Google
Citado por Google -
 Similares en
SciELO
Similares en
SciELO -
 Similares en Google
Similares en Google
Compartir
Anales de Psicología
versión On-line ISSN 1695-2294versión impresa ISSN 0212-9728
Anal. Psicol. vol.32 no.3 Murcia oct. 2016
https://dx.doi.org/10.6018/analesps.32.3.259401
Identification of Verbal and Mathematical Talent: The Relevance of "Out of Level' Measurement
Identificación del Talento Verbal y Matemático: La relevancia de la medida "out of level"
Javier Tourón1 and Marta Tourón2
1 Universidad Internacional de la Rioja (UNIR) (Spain).
2 Especialista en Altas capacidades (Spain).
ABSTRACT
This study has two mam objectives. First one to carry out a conceptual review of the literature together with the work done in Spain by the authors about the identification model known in the international literature as Talent Search model or concept. This model created by J. C. Stanley in the early 70s has led to a huge development in the identification of verbal and mathematical talent of young people, in order to provide the appropriate educational provision their ability needs. Far from being an American model, in this paper we show, and this is the second objective, through data from several years of implementation of the model in Spain, that it can be considered a universal model, based among others in the principle of above or out of level measurement. Using this above level measurement, we can adequately discriminate the diverse ability of the students tested, that when measured alone with in level testing, is masked due to lack difficulty and discrimination of the tests used. Some suggestions for large-scale use of these procedures in schools are provided.
Key words: Identification, Verbal Talent, Mathematical Talent, Talent Search, Centre for Talented Youth, SCAT, Above Level Testing.
RESUMEN
El presente estudio tiene dos objetivos principales. Por una parte, se ofrece una revisión conceptual de la literatura junto con los trabajos realizados en España por los autores acerca del modelo de identificación del talento, conocido en la literatura internacional como Talent Search model o Talent Search concept. Este modelo creado por J.C. Stanley en los EE.UU. a principios de los 70 ha dado lugar a un inmenso desarrollo en la detección del talento verbal y matemático de los jóvenes, con el objeto de ofrecerles ayudas educativas adecuadas a su capacidad. Lejos de ser un modelo americano, en este trabajo mostramos, y este es el segundo objetivo, a través de los datos de varios años de aplicación del modelo en España, que puede considerarse un modelo universal que, apoyado entre otros en el principio de la medición por encima de nivel (above or out of level), permite discriminar adecuadamente la capacidad diferencial de los alumnos que, al ser medidos solo con pruebas de nivel, su verdadera capacidad queda enmascarada. Se ofrecen algunas sugerencias para el uso a gran escala de estos procedimientos en las escuelas.
Palabras clave: Identificación, Talento Verbal, Talento Matemático, Talent Search, Center for Talented Youth, SCAT, Above Level Testing.
Introduction: preliminary assumptions
In recent years we have witnessed a remarkable evolution over the focus and conceptualisation of high abilities, new initiatives that try to unite high capacity, talent, creativity, innovation and excellence appear. Nobody seems to doubt, at least theoretically, of the magnitude of the education systems as mechanisms of intervention and social development. It is less clear, however, that the concerns of many are aligned with the development of talent and the potential of many young people in our countries, deliberately or not ignoring the importance of this for the social construction and human freedom.
The educational process becomes the key to transform the natural abilities into systematically developed ones in Gagné's terminology. Only a few still hesitate to make clear that we are talking about a process of development; the postulates that understand that high ability is an attribute or state of being, that some are and that some not, are far away. This "fixed" position of intelligence and capability has resulted in a clear change of paradigm, in which the ability is the starting point and the development of talent in a domain or more, the arrival point that, eventually, can lead to excellence and even to eminence (See Renzulli and Geasser, 2015; Pfeiffer, 2015; Gagné, 2015; Olzewski-Kubilius, Subotnik & Worrell, 2015). Obviously, this process must be systematically scheduled. In other words, skills or abilities in a field or more will not become more "operational" (so to speak) naturally; it is the scheduled and systematic training which will make these capabilities contribute to the development of skills in a given area.
Therefore, the level of competence and skill, of expertise if you will, in a field of knowledge, will be the result of the projection of capacity in this field, being the efficiency on it the effect of the educational development. Thus, to be competent in a field, the appropriate skills are needed, but also a set of resources and appropriate intervention programs and a no small dose of work, effort and motivation for achievement and excellence, that is to say, of the non cognitive factors (Dweck, 2008; Dukworth & Gross, 2014, Ericsson, 2007).
It is crucial to understand, then, that talent is based on (partly inherited) personal circumstances to be projected (at best) in various fields of human activity. But it is also essential to understand that talent does not develop spontaneously. Therefore, the ability should be seen as potential, talent as an efficiency to a greater or lesser extent, so that talent is the result of applying personal effort, the will, the motivation, the development of which initially are only dubious potentialities (Cfr. Gagné, 2009, 2015).
The role of intervention programs will be to achieve that the potential turns into performance. That the potentialities turn into competences1. Thus, it is easy to understand that it is not the same to have a high capacity for quantitative reasoning and being a good mathematician and just have great knowledge (and skills) in the field of Mathematics. The ability is necessary; it is true, but also the work and the help to put it at the service of advanced achievements in a given field.
This, as it is understood, has first order educational consequences because of what is stated above. We can state inmediately that all the talent that is not cultivated may be lost, but for being cultivated it must be identified first. Thus, identification and intervention become two axes of talent development. It seems unnecessary to insist that education must ensure that the efficiency of people is equated with their potential. It is not about being the first of the class, or be above this or that evaluative reference. It is simply about enable each person to develop optimally.
If schools were truly adaptive and respond therefore individually to the needs of each student, to identify the most capable students would not be an issue of particular importance because, in one way or another, everyone should be assessed on the critical variables for learning, and in their level of knowledge and skills in various areas (See Tourón, 2009; Stanley, 2001).
A school based on age and not on competence, focusing on the activity of the teacher and not on the student's role, it is a school that is unable to meet the personal differences (see Touron and Santiago, 2013, for a more detailed analysis on this subject).
In this context it is clear that all schools should have procedures to systematically evaluate and regulate the potential of all students. It is not enough a reactive system that, at best, offers some help, at some moment, in some courses or educational levels. It is necessary to move in a different direction seriously, to a proactive system in which the rule is personalization and therefore the goal is the development of talent (Touron and Santiago, 2015).
The purpose of this paper is twofold. On the one hand, briefly discuss the theoretical aspects of the talent identification model that more extension and more success has had on the world and on the other, provide the results of its implementation and operation in Spain in the last fifteen years.
Talent Search Model: a bit of history
Various names have been used to refer to the Talent Search. In a monograph of High Ability Studies, a few years ago, we used the expression: The CTY Model, also known as Talent Search Model, or SMPY model (Study of Mathematically Precocious Youth) (See Tourón, 2005). Any of these names can be considered reasonably equivalent, although the origin of these is SMPY, which is the name of the project that Professor Stanley begins in Baltimore in the early 70s and continues its 50-year longitudinal study. SMPY is currently run by Benbow and Lubinski at Vanderbilt University2. Even though, as Benbow and Lubinski noted, the acronym SPMY is a bit inadequate as it not only deals with the mathematical dimension, but also with verbal and is not aimed only at young people, the model has been extended to children and adults who are currently part of the cohorts analyzed in the longitudinal study (See Lubinski and Benbow, 2006). The longitudinal study begun in the seventies and was designed for fifty years and is approaching its final stage (Lubinski, Benbow & Stoeger, 2014).
The model exceeded all initial expectations of its creator, because after all the years there are many universities that have implemented it in the US and elsewhere, and several million students have benefited from some process of identification, program or services offered by the most diverse institutions (See Tourón, 2005, 2010).
SMPY model cannot be understood in its extension if you do not understand its creator, Professor Julian Stanley. His interest in high ability students began with the study of intelligence and tests as instruments to measure it; not in vain he was already a renowned methodologist when he began his adventure in the world of talent development3. There is no doubt that there is an "informal" preliminary work that would become the germ of a model that over time has been directly or indirectly influencing many current forms of guiding the work of the more able students. He describes it in numerous articles and essays (for example: Stanley, 1974; Stanley, 1977; Stanley, 1989; Stanley, 1990a; Stanley, 1991; Stanley, 1996; Stanley and Brandt, 1981; Stanley and Benbow, 1983; Stanley and George, 1978).
There is a feeling that is perceived in the writings of Professor Stanley. It's like having achieved a goal which, without being predetermined from the beginning, is accomplished at the end of a long way. It's like a combination of circumstances that conclude in a hidden purpose that come to light after years of work. Stanley himself explains how, when he had the first case of a boy with an extraordinary ability, immersed in work, he postponed his identification a few months. Then, after seeing the student and study the case, he will state, "thereafter, my life and my career were not the same" (Stanley, 1996; Stanley, 2005)4.
It was the summer of 1968 and after a series of considerations Stanley decided that the student should take the College Board Scholastic Assessment Test (SAT)5, a test which was then used, and that it is currently used for admission in American universities. There is no doubt that behind this, apparently elemental, idea was Leta Hollingworth, who had already sensed this as valid, and that was read several times by Professor Stanley in his formation years. Bates joined Johns Hopkins at the age of 13, as his intellectual ability joined a suitable personality and sociability. Bates graduated in 1973 with 17 years and at 24 he obtained a doctorate in computer science after a disturbing journey through education (Stanley, 1991; Stanley and Benbow, 1986). It can be considered the first "radical accelerant" as Stanley calls him (Stanley, 1974).
There is no doubt that these original ideas, the strong personality of Professor Stanley, and continuous, demanding and precise research of SMPY, have resulted in a model, that joining identification and treatment has helped to strengthen the education of high ability students.
This way, previous experiences, and the genuine interest of Professor Stanley for young people with intellectual talent, encouraged him to embark on a new line of research. In 1970 he obtained from the Spencer Foundation a generous grant for five years, which is finally renewed until 1984 (Stanley, 1996).
In September 1971 the SMPY (Study of Mathematically Precocious Youth), whose initial aim was to identify and educate high school students who were precocious in Mathematics (Benbow, 1986)6 officially begins. In the words of Lubinski, Benbow and Sanders (1993), the original interest of SMPY was identify adolescents who had exceptional intellectual abilities, and then find out the factors that contribute to their optimal educational development.
To achieve the first objective, it was decided to make an annual talent search; from the first year of implementation, it has been one of the key elements of the model.
For this, assessment above level7 was used, since, according to previous experience, it seemed an appropriate method of identification. This test would administer to students in 7th and 8th (1st and 2nd of Secondary Education) who were in the top 5% of mathematical performance, judged from another test of standardized performance than they had been administer to at their school, something relatively common in the US.
The first Talent Search was conducted at Johns Hopkins University, on March 4, 1972. A total of 450 students from 7th and 8th (12-13 years) in the metropolitan area of Baltimore did tests of Mathematics and Science of a great difficulty for their age. Many of them had scores that were around 690 points, as the maximum that can be achieved with this test was 800. Clearly, says Keating (1974) when performance or aptitude tests are used to assess the ability of these students, their scores are comparable to those of students who are going to start college. A search was followed by others, in January 1973 and 1974, in December 1976, January 1978 and January 1979 and so on until the present. In the school year 1982/83 the Talent Search formed a circuit throughout the United States that has grown and continues to be very effective thanks to all the accumulated experience over decades.
Table 1 presents briefly some data on the evolution of this model that can indicate how its growth has been and what have been their innovations. The idea was to find young people with special talents that could be helped so they could move through the curriculum, educationally speaking, faster and further. According to Stanley, Terman and Pressey8 had already provided great information against the prevailing stereotypes, and had affirmed the need to take appropriate educational measures with the more able students. However, it is not possible to use these measures without an accurate knowledge of young people who need them. Therefore, an effective identification was clearly the first step.
We should now ask, to whom is identification addressed? High ability students, but what it is meant by high ability students? The answer can be summed up in one word: precocity
Some principles about Talent Search
The SMPY (CTY we would say today) focuses on students who are precocious in math and verbal areas, while efforts made to identify and enhance other areas of talent are recognized and encouraged. The areas that CTY addresses to are central in the architecture of all school learning and good precursors of the academic potential of students. They are also easily assessable.
Secondly it is important to note that the SMPY does not use the term "gifted" to refer to the students he works with. The word gifted, it is stated, "should be reserved for those who have made significant contributions to the advancement of knowledge and practice" (CTY, 1995, p. VI). Thus, the term gifted should be understood more as a point of arrival than of departure.
Young people with greater potential or ability are characterized by their precocity, because they show a, sometimes exceptional, progress in relation to what is proper to their age. It is precisely this precocity which requires a differentiated educational treatment. (See e. g. Keating 1976; Benbow, 1986; Keating and Stanley, 1972). In sum, the SMPY model sees high ability as synonymous with precocity (Benbow, 1991), relying on multiple investigations into the matter (Jackson and Butterfield, 1986; Keating and Schaefer, 1975; Brody and Stanley, 2005; Stanley, 2005).
There are some principles that, as we are seeing, it is the result of practice and not of a previous theoretical elaboration, which does not mean in any way that is not perfectly based on well-defined psychoeducational principles (see Brody and Stanley, 2005).
The SMPY has a set of principles on which it bases its action, both in relation to the processes of identification and on the implementation of intervention programs that can be seen in Brody (1999, 2009a, 2009b).
Basically these principles recognize the differences in ability that require differentiated educational treatment, but for this to be possible it is necessary to identify such differences. This is precisely the focus of the Talent Search.
In any case we must understand that the CTY model born to help in the intellectual, academic, social and emotional development, personal in short, of the students and this effort is not intended to compete with the school, because the activities proposed have after-school and extracurricular character. His intention was to supplement and complement classroom instruction, not supplant, invade or criticize it (Stanley, 2005).
The CTY model is essentially linked to the educational action, but to intervene it is necessary to know who has to be the receptors of the intervention, who are the students whose potential is not adequately stimulated. Thus, the first is the discovery of talent, which is conducted through the talent search, which are carried out systematically every year
But it is also necessary to carry out the description of the different profiles of students' abilities, interests, their strengths and weaknesses, their degree of talent, requiring various educational planning. We have already noted above that talent varies widely, even in highly selected groups. Likewise it will be necessary to adapt the educational response depending not on whether a student has a talent or ability or not above a given level (the absurd may be or may not be), but how much is above that level. Or what is the same, how exceptional is their ability.
The mathematical precocity, along with verbal, are one of the key concepts in this model. Benbow (1986) notes that, while it is common for researchers to define and conceptualize high capacity when they start working in this area, the SMPY has not been overly focused on this. The reasons are practical. It seems more effective, according to the results of years of research, the option to identify students who are brilliant at math, or other area, and organize the environment to help them learn as well as possible, than the option to avoid any intervention until the concept is clearly defined.
In this sense, Keating and Stanley (1972) affirm, referring to mathematical-scientific area, that the objective of the SMPY is not locate each of the brightest students and "push" towards mathematics or science to make them "scientists." First, it is unlikely to be possible to do that even if you want. Second, the interest of the project is to help and assist the talent, employing for that the tests in the identification process.
On the other hand, it is not to create a set of unique programs for exceptionally bright students, but to take advantage of the resources already available, but considering that the flexibility and individualization, personalization we would say today, are the principles that should guide the work with these students. In this sense, Stanley and George (1978) also state that the SMPY model is longitudinal and it is developing, but not "genetic". It does not explore the origins of high ability that is possessed at 12/13 years, however, makes a great effort to take advantage of the current early development of the student, through appropriate educational intervention.
Therefore, the operational definition of talent that since its origin is used by the SMPY is high score on the SAT (School Assessment Test) at an early age. Given that the SAT is a test that is used for identification but above level (as will be explained later), this means that the SMPY sees high ability as synonymous with precocity, as we have noted. Furthermore, the purpose of SMPY was not only the identification, it searched and searches also to provide not only the most appropriate educative help, not only to the type of talent but also to its range or level. So for Stanley (1991) identification and description were insufficient, they should help the most able young people to materialize its full potential with the most appropriate educational measures.
The SMPY believes that for the optimal development of talent, not only the individual must possess certain personal attributes critical for the success and satisfaction in his vocational choice, but he must also be given the opportunity to develop them in an educational environment of appropriate learning. All components are therefore vital. So, Benbow and Lubinski (1997) claim that, "the practical implications of SMPY are, first, to identify appropriate educational and vocational means for each particular individual and then try to organize educational interventions according to their abilities and specific needs" (p. 158).
It can be considered that Leta Hollingworth, a pioneer in the study of the ablest young people, is one of the clearest influences on SMPY model. References to this author are common in the writings of Professor Stanley. Hollingworth had 32 when he was born, and at the death of the author he was 21 and was his third year teaching Science and Mathematics in a secondary school. Her work and her example, he says, "have had a profound effect on my professional life" (Stanley, 1990b, p. 168).
The first time that Hollingworth used a test above level was in 1916, the year that she doctorate (see Stanley, 1990b). She wrote her first article on children's high ability in 1917. Since 1922, she worked deeper into the issue of high ability, considering as such to those subjects who got an IQ of 180 or higher on the review that Terman did in 1916 of the Binet-Simon scale. Hollingworth clearly saw the need to evaluate with a more difficult test for those children with a higher capacity.
Discovery and description through the Talent Search
It can be said, without any doubt, that the identification is a crucial element in the SMPY, but only if it is understood as a prelude to the remaining phases of the model. That is, the operational nature that is reflected in the way to approach the concept of high capacity indicates the need to find those young people with an extremely good verbal and mathematical reasoning, and then provide them specific support that may need.
While at first the Talent Search was seen as an identification mechanism, where tests identified those children who stood out for his talent in math or verbal areas, and it selected them to participate in special programs, then this idea will reconceptualize, and Talent Search begins to be understood as a diagnostic tool to discover areas and levels of ability, but within a population that is already considered of high ability, and offers students different educational methods that are appropriate to their pace of learning (Olszewski-Kubilius, 1998).
What the model shows is that two students, who obtain the same score in a suitable test for their level, obtain very different scores on a test that is above level, as shown in Figure 1. This means that if a teacher is based on the scores students get in a test of in level, will place both on the same level and will offer the same educational programs. However, if it is based on test scores that are above level, programs, strategies and resources used with each of them will be different. Both are high-ability, without doubt, but maybe one is enough for an enrichment program, while for the other will be more convenient an advanced math program, with a faster pace than usual. In other words, the "out of level" is to eliminate or mitigate the ceiling effect that the "in level" measure can have for the ablest students. The lack of difficulty of the test for these turns into lack of discrimination, so apparently different students are seen as equals; that is, they get the same score when its capabilities are clearly different. This effect is more pronounced the closer the student's ability is to the higher test score. This effect is universal and not only applies to tests used in this model, so it should be considered in any process of identification, individual or group based.
Goldstein, Stocking and Godfrey (1999) show that the basis of the identification of SMPY is that standardized tests traditionally used to evaluate the academic performance of students at school age, are considered to have a ceiling that is too low to identify those students whose talents are so rare that they need and deserve special educational opportunities. Get a score in the 99th percentile on a test, for example the California Achievement Test, is a remarkable performance, certainly, but the 1% of students who are in this select group still represent a very broad range of ability. As Benbow and Lubinski (1993) note, the top 1% of individuals in most distributions covers such a wide range as the one that ranges from the lower 2% to the 2% higher. In terms of IQ, the range of scores for students who are in the top 1% (135 to 200) is as broad as the range of scores between the 2nd percentile and the 98th (66 to 134). It is therefore necessary to use above-level tests with students who obtain higher scores on tests of their level. Thus the opportunity to demonstrate the full extent of their capacities is obtained.
In sum, the novelty of this model, with regard to identification, occurs, in our view, in two ways: a) on the one hand, the evaluation out of level, that is, the use of tests of higher levels than the age of the students tested for identification, and 2) in the description from the scores obtained by students in the tests, because their profile and characteristics are analyzed, along with their scores and it is determined the most appropriate way to intervene in each case.
The identification process
In the first phase students who may participate in the Talent Search11 are selected. They must have reached the top 95th or 97th percentile on a standardized aptitude or performance test that can be administered generally within the normal evaluation process of their schools. The CTY itself offers a wide list of tests that allow qualifying for the Talent Search11. This percentile has varied slightly over the years. But these details are not important now.
For example, between 1972, when it was held the first Talent Search, and 1978, a total of 9,927 students between 12 and 14 years have participated. The percentage stipulated to move to the next phase of the process may vary depending on the year, but is always between the 2 and 5% (Benbow and Lubinski, 1997).
Why the top 3 or 5%? Goldstein, Stocking and Godfrey (1999) from the data obtained in their Talent Search at Duke University show that there is a big difference in SAT scores among students who were at the 99th percentile of a standardized test of their age level and those who were at the 98 or 97th percentile of the test (see Table 2).
That is, below the 3-4% almost no student gets a score above 500 on the SAT, so it is reasonable to conclude that a cut-off point below this would not lead to satisfactory results and on the other hand, it would produce situations of 10 frustration with students (Goldstein, Stocking and Godfrey, 1999).
In any case, most universities do not set as a prerequisite the identification via Talent Search to participate later in the programs and courses they offer. Many students take the SAT or ACT on their own, presenting their scores, and without the needing to take the two phases of identification. In fact, as claimed by Goldstein, Stocking and Godfrey (1999), "TIP12, and other programs like this, do not seek to exclude pupils using the Talent Search, but will seek to identify students who can benefit the most from programs they are offered. Therefore, it seems appropriate to continue using the 97th percentile as cut-off point, but considering that this is a guide, not a barrier" (p.145).
The second phase (above or out of level testing) of identification is one of the most characteristic features of the SMPY model. The idea of using tests above level is not new, as we noted, but their use in a systematic and annual manner to identify high ability students is. The main advantage is that it allows to discover the intellectual differences that occur between the ablest students, who often are concealed when conventional tests, which usually have poor discriminative ability for them, are used. These individual differences among students are educational and psychologically very significant and are of great importance to plan and structure the educational intervention, especially if it comes to the use of accelerative resources.
Benbow (1992) states that the differences in the academic performance of young people who are in the top 1% are very noticeable. In a period of 10 years, between 13 and 23 years, the academic performance of students who were at the upper quarter of the top 1% in mathematical ability was much more spectacular than that of those who were at the lower quarter of the top 1%, which also had a very high performance. Therefore, Benbow and Lubinski (1997) say that, "the different expectations for students who are in this range, which involves IQ scores between 135 and 200, are justified and should be established" (p. 159).
Out of level assessment in the Talent Search, allows students themselves to know what their strengths and weaknesses are with respect to the more characteristic intellectual abilities of academic excellence: verbal and mathematical reasoning.
Although the SAT is the test that has been used traditionally in the model, currently, and since the groups that can participate in the Talent Search have increased, various tests are used. Although it would be interesting to do it, it does seem neither the time nor the opportunity to deepen into this issue, extending this study more than is reasonably necessary.
Method
Implementation of the Talent Search in Spain
We will briefly mention the implementation of the principles of Talent Search in Spain (descriptions of the process that we will not comment here for lack of space can be found in Reyero and Touron, 2003; Tourón and Tourón, 2006, 2011).
In Spain, as in many other European countries is not common that standardized performance tests that have national or regional standards are used, so it is necessary to select students of that top 3 or 5% in a different way as it is made in the USA. Here come the evaluations of teachers, parents, self-nominations, nominations of colleagues, etc.
In the case that we are going to analyze we use the SCAT test, The School and College Ability Test, originally developed by ETS (Princeton) in the 70s and currently owned by CTY (Johns Hopkins University). This test, with three difficulty levels, is designed to measure verbal and quantitative ability of students from 3rd course of Primary Education and 2nd year of Upper Secondary Education. The first level, called elementary, is administered to students from 3rd to 5th grades in Primary; the intermediate to 6th grade students and 1st and 2nd of Secondary Education (here we called them 7th and 8th); the advanced level is administered to students in grades 9th to 12th (4th of Secondary Education to 2nd year of Upper Secondary Education). Scores vary in each section between 0 and 50.
This test was validated by the first author of this work, a process that is reported in other studies (Brody, L. E., Stanley, J. C.; Barnett, L. B.; Gilheany, S.; Tourón, J. & Pyryt, M. C. 2001; Tourón 2001; Tourón and Reyero, 2002, 2003; Tourón, Tourón & Silvero, 2005).
Since in our country standardized perfomance test are not used, as we just noted, we use the same SCAT test for both processes 'in level' and 'out of level'. Thus the process is more long and complex, but it is the best way to select those students for whom the measure 'in level' could be producing a ceiling effect.
As a standard way we select those students that in phase 'in level' obtained scores that place them in the PC 95 or higher. To determine the level of the ability of these students we re-evaluate them with different levels of SCAT battery and we use the scales of comparison as set out in Table 3.
The courses of the Compulsory Secondary Education and Upper Secondary Education will be called consecutively 7th to 12th, for convenience and approximation with the American system.
As can be seen, once students have passed the 95th percentile in the phase 'in level', they are evaluated with the level of the battery indicated in the table. There is an exception which is that younger students (3rd year of Primary) who are not reevaluated, do not perform a new test in the phase 'out of level', but their scores in the first phase are directly compared with the scale of the two years older students, to compare above level.
The remaining students are assessed with the level of the next higher battery thanthe one used in phase 'in level', but it is also important to note that their results are compared with those obtained by students between 2 and 3 years older than them, in this way we can see the extent of his verbal and mathematical skills compared with older students.
As we noted above, it is necessary to recognize the intellectual differences among the most capable students who, far from being a homogeneous group, as some have come to believe naively, they have outstanding differences and diverse educational needs.
The cohorts of this study
In this paper we will bring together all the data from the last fifteen years that we will divide into two big cohorts. The first consists of students from third grade of Primary (N = 2294) who come from a census evaluation in a Spanish autonomous community, cohort 2 consists of students from 4th of Primary to 2nd of Secundary Education (N = 759). All data have been the result of a student assessment in the indicated levels between 2002 and 2014. They are not, and therefore, not intended to be a sample of a population. They are a large set of evaluations that we use here to show the principles of the model and thus serve as contrast of itself. Table 4 shows the data of the number of students per grade.
Data analysis
The reason for dividing the data into two cohorts is that, as noted above, the students of 3rd do not materially perform a new test, but its phase "out of level" is done simply by comparing their scores with the scale of the older students (see Table 4).
The results of each cohort have been analyzed in its most useful descriptive statistics and compared the percentile scores in the phase in level with the phase out of level, calculating the function (linear or quadratic) that best fits to the relationship between the scores.
Moreover we have made the scatter diagram in order to verify the relationship between percentiles in each stage for pupils that in the first (in level) they had a 95th percentile or higher. We have also calculated the function relating both percentiles scores and correlation coefficients between them.
Results
a) Cohort 1
In Table 5 we collect descriptive statistics for cohort 1 and that belong to a group of students from 3rd grade of Primary, almost all the students in that educational level in the community to which they belong. As shown in Figure 2, the dispersion of scores covers virtually the whole scale, being the maximum verbal score 47 (remember that the maximum is 50) and maximum mathematics score 43.
This reflects an obvious fact, but often ignored: the enormous diversity that is present in any measured ability, in students of the same chronological age. As an example, we can say that in these distributions there are 35 students who obtain verbal results that are at the 99th percentile (41 points), while in mathematics section there are 28 students who obtain equal to 37 or higher, which is also equivalent to 99th percentile We can now formulate some questions: is the ability of these students properly stimulated by regular school programs?, is its ability a guarantee that their learning speed will be at least diverse of the students with much lower scores?, will be identified by their teachers so that they can receive an instruction with the appropriate level of challenge, the only way to ensure that their ability will be developed? And finally, is this the limit of its ability or will be the test presenting a ceiling effect for them?
We could make the same argument about any other percentile, such as 90 or 95, for example, to come to the same conclusion. An inspection of the Figure 2 will be enough to clearly see the fact that we note. Students have very different scores indicating different abilities and therefore educational needs will also be different.
Another question that will represent the heart of the model we are studying must be still answered. A 3rd grade student with a determined score on their in level testing, when compared with the level of performance of 5th grade students, two years older, how exceptional or outstanding is?
Figures 3 and 4 try to answer this question, by the combination of the 'in level' scores with out of level' ones13. A quick inspection of them allow us to see that there is a significant quadratic gradient (see figure) pointing patently that as scores 'in level' increase in value, scores out of level' do exponentially. This effect is somewhat increased by the fact that, as noted (see note 14), a score 'in level' is assigned to a corresponding score out of level' which does not happen in the other cases whose score 'out of level' comes from the administration of a new test level, which increases the variability of possible student responses. It is, however, obvious that there is a clear trend that intensifies when the score is higher, so the ceiling effect is more pronounced the more we approach the limit of the test.
Correlations between percentiles 'in level' and 'out of level' calculated from the coefficient tau-b of Kendal for this set of data in this cohort were significant for values of 0.96 for verbal dimension and 0.94 for mathematical dimension, a fact that is reflected in the figures.
b) Cohort 2
This group consists of data of the grades 4th to 8th Primary (2nd of Secondary Education). The effect of the dispersion of scores is, as expected, similar to that we see in students in the 3th grade, as shown in Table 6 and Figure 5. A careful observation of the distributions of the frequency of the various grades in either of the SCAT sections reveals that there are students with very high scores, close to the upper limit of the scale. We can ask the same questions that we made about third grade students. Is it possible to help them educationally in their needs without differentiating the curriculum or their speed of development or depth? It does not seem possible. But this is where the Talent Search model shows its full potential. When these students obtain scores that place them in the 95th percentile in its measures 'in level' (it is not an inflexible cut-off score), make a new assessment "out of level" according to what is stated in Table 3, being compared as we have reiterated with students several years older than them. What happens then?
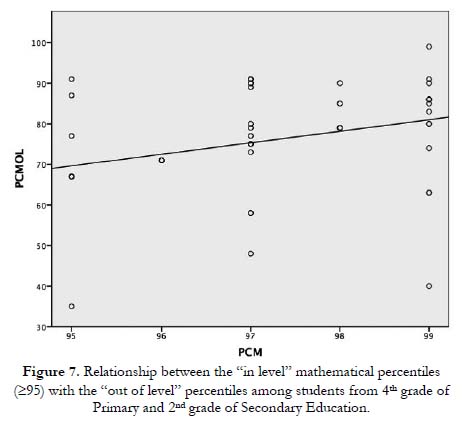
Figures 6 and 7 represent the relationship between the scores 'in level' and 'out of level' for students in this cohort. Each point on the graph may represent more than one pair of values. As shown, there is a clear trend in the sense that when the percentile 'in level' increases the percentile 'out of level' does it too. At the same time, it is clear also that the same percentile 'in level' leads to very different "out of level" results, showing that the ceiling effect is not, in any way, neither equal nor uniform for all students. To provide more clarity on this point, in Table 7 we collect empirical minimum and maximum values obtained by the students tested in scores "out of level" for each of the percentiles 'in level' that we are considering.
As we see the variability in the case of verbal section is slightly smaller when the percentile 'in level' increases, or what is the same, the ceiling effect grows when scores do. The differences in the case of mathematical or quantitative section are even more pronounced, as well as for a student with an 'in level' 95 percentile, the 'out of level' percentile has resulted in some cases in 35, which does not reflect apparent ceiling effect, but in other case it was 91, which seems to show it. Here we also see that although the score 'in level' is high (99), the ceiling effect (99) and the lack of it (40), may be present. Naturally it is not possible to know in advance whether the ceiling effect is present in a particular student, making it even more important to check with measures "out of level" but not by the fact itself, but because what we want is to know the level of the student's ability, as it seems clear, that the higher it is, the most outstanding shall be the educational measures taken.
Finally, the correlations- estimated through the Kendal tau-b coefficient- between "in level" and 'out of level' percentiles in the case of the set of data in cohort 2 also have been significant, with values of 0.60 for the verbal section and 0.55 for mathematics. These values are lower than in cohort 1 because students take a new test, so there are many other factors that influence the results that may be obtained. However the relationship is fairly consistent and basically coincides with previous studies mentioned above.
Conclusions
To complete this paper in which we summarize data from fifteen years of assessments with SCAT applying the strategy of the Talent Search model, we want to set some conclusions derived from what has been presented so far.
The Talent Search model developed by Professor Stanley in the early 70s in the US, has resulted as an effective tool in the detection of young people with high ability. Originally focused on the use of the SAT, their principles of measurement out of level (out or above level) have been applied to millions of children and youth not just American but from many other countries, also in Spain, as we have shown in this paper. Today it is a common practice in many other universities apart from Johns Hopkins, constituting an effective tool in the detection of verbal and mathematical ability, as the two main areas of development of academic talent, in order to provide these students with educational opportunities that give them the appropriate level of challenge to develop optimally their talent. That was precisely the aim of the Professor Stanley when he created its model, to encourage all students so they could realize their full potential, which is perfectly in line with the current general approach that considers ability (high ability) as an evolutionary process, as we noted at the beginning of these pages.
Naturally the "high ability" does not end with the academic dimension, and the model does not want to suggest such a thing. What it is wanted to be highlighted is that ability requires intervention to become talent (competence) in different domains of human activity, so having systematic and parsimonious tools, easy to implement on a large scale, is highly importance. It may be noted, as we did on another occasion (see Tourón and Tourón, 2011), that the Talent Search is not, in any way, an American model, because as we have seen, its principles are cross-cultural.
If we accept, according to the most relevant authors in the field of high abilities (See Geasser & Renzulli, 2015; Pfeiffer, 2015; Gagné, 2015; Olzewski-Kubilius, Subotnik & Worrell, 2015, among many others) that ability needs an adequate process for developing and implementing a given domain, it makes no sense to talk about "giftedness" as a real, physical, construct, as a state of being, but as a developing capacity, which requires other environmental and intrapersonal non-cognitive dimensions to produce excellent results and in some cases, eminent. Therefore, to have processes of identification of the variables that in each case are relevant for the intervention or educational services that are going to be promoted (ideally, but not only, within school) guarantees that talent can emerge. With no personal attention to the needs of each student, this may remain a chimera.
Moreover, referring to the data analyzed here we emphasize that:
a) The diversity of the scores obtained by the students on the measures of verbal and quantitative (mathematical) ability, in the SCAT, as they do in any other test, are huge, so it makes no sense to consider students in their educational needs according of their age, that in the school we have is the same, but their competence which is, as it is clear, very diverse. Ignoring these differences is unwise if we want to optimize the development of the potential of each student.
b) When students obtain high scores on measures that are administer to them according to their age, in particular to the 95th percentile or higher, their ability can easily be underestimated, so it becomes necessary to use above level measures. That is, measures with similar tests that have been designed for students older than them.
c) When carrying out this measurement above level ("of level") it is showed indeed that 'in level'tests make that students of various abilities receive the same scores, due to lack of difficulty and therefore of discrimination in the tests.
d) 'Out of level' measurements are effective to discriminate between students of various abilities that are measured as equal in the phase 'in level', allowing to know not only which students have talent or not, but to estimate the degree of it, what eventually will facilitate to provide them educational measures with an adequate level of intellectual challenge. Correlations between the scores obtained by students in both phases corroborate this.
e) The SCAT, normed by the authors in Spain, particularly in the region of Navarra, has proved to be an excellent tool to detect not only the talent of the students tested, but to elucidate, in most cases, the degree of it, reevaluating students as we have explained.
It is, in our view, of the highest importance that the educational system, every school in particular, determine the capacity of their students and use a program that allows personalize their learning paths, this will be a guarantee that the school really bets for talent development, beyond labels, rigid cut-off points, inflexible curricula and conceptions of education which are typical of past times.
1 The OECD defines "Competence" as "a combination of skills, knowledge and attitudes that a person possesses" (OECD, 2005).
2 All the information and a large number of online publications can be found at: https://my.vanderbilt.edu/smpy/
3 Their works on the validity of the experimental design with D. Campbell or statistical texts with G. Glass are famous.
4 This student was Joseph Bates Louis Middleton who followed Jonathan Edwards. An analysis of the historical path of the SMPY can be found, for example in Reyero and Tourón (2003).
5 The SAT (Scholastic Aptitude Test) is a test that prepares the Educational Testing Service (ETS, Princeton) for the College Board directed to the students of grade 11 and 12 (high school seniors), which is required for the admission to many US universities. The acronym is maintained, but has changed its meaning. The test is called, since March 1993, Scholastic Assessment Test. The decision to change the name was taken by the Council of the College Board, according to the report by David Gardner and Derek Bok, former presidents of Harvard and California, respectively. According to the report, the test "by its nature and purpose" much more measured than the term aptitude might suggest. The previous name was used from 1926 (From Tourón, Peralta and Repáraz, 1998, p. 88). Currently it has undergone a major renovation which can be seen on College Board page: https://collegereadiness.collegeboard.org/sat-suite-assessments
6 The SMPY focused, in its beginnings, in the mathematical talent, since the first investigations of Stanley deepened in features and procedures of youth development with an extraordinary capacity of mathematical reasoning. However, over time, he joined the verbal mathematical talent, considering both key in academia.
7 In the specialized literature this evaluation is called "out of level" or even "above level".
8 Pressey (1949) postulated freedom for the more able students so they can tour the entire school system as quickly as they need. This author is, with Hollingworth and Terman an emblematic figure in the study of high intelectual ability. According to Stanley, Pressey went a step further in relation to the previous two, since it showed that the alleged negativity of educational acceleration was not.
9 Currently there is an association of schools that implement the model. It can be checked at: http://cty.jhu.edu/international/about/ For further information about colleges that implement the model with some own variants can be checked the High Ability Studies monograph edited by Tourón (2005), or Reyero and Tourón (2003).
10 Although Talent Search is usually used to refer to the entire identification process comprising both the evaluation "in level" as the evaluation "out of level", it is not uncommon to find in the literature the term Talent Search to refer only to the second part.
11 Interested readers can visit: http://cty.jhu.edu/ts/tests.html
12 This program is the equivalent of CTY, but is developed at Duke University.
13 The test can also calculate a total score (sum of the two sections), but here we will not consider it.
14 It is worth remembering that these students are assigned scores (no action) directly from the 5th scale, because these students are too young, with few exceptions, to face the intermediate level of SCAT. That is in phase 'out of level' is assigned the percentile that corresponds to their raw score on the scale of 5 degrees.
References
1. Benbow, C. P. (1986). SMPY's 15 years of Research in a Nutshell. In J. Van Tassel Baska, (Ed.), The Richardson Study: A Catalyst for Policy Change in Gifted Education. Evanston, IL: Center for Talent Development (Northwestern University). [ Links ]
2. Benbow, C. P. (1991). Mathematically Talented Children: Can Acceleration Meet Their Educational Needs? En N. Colangelo& G. A. Davis (Eds.), Handbook of Gifted Education. Boston: Allyn and Bacon. [ Links ]
3. Benbow, C. P. (1992). Academic Achievement in Mathematics and Science of Students between Ages 13 and 23: Are there Differences among Students in the Top one Percent of Mathematical Ability? Journal of Educational Psychology, 84(1), 51-61. [ Links ]
4. Benbow, C. P. & Lubinski, D. (1993). Psychological Profiles of the Mathematically Talented: Some Sex Differences and Evidence Supporting Their Biological Basis. In G. R. Bock & K. Ackrill (Eds.), The Origins and Development of High Ability. New York: John Wiley and Sons. [ Links ]
5. Benbow, C. P. &Lubinski, D. (1997). Intellectually Talented Children: How Can We Best Meet Their Needs? In N. Colangelo & G. A. Davis (Eds.), Handbook of Gifted Education. Boston: Allyn and Bacon. [ Links ]
6. Brody, L. E. (1999). The Talent Searches: Counselling and Mentoring Activities. In N. Colangelo & S. G. Assouline (Eds.), Talent Development III. Proceedings from the 1995 Henry B. and Jocelyn Wallace National Research Symposium on Talent Development. Scottsdale, Arizona: Gifted Psychology Press. [ Links ]
7. Brody, L. E. (2009a). Personalized Programs for Talent Development: The Johns Hopkins Model for Meeting Individual Needs. In B. MacFarlane & T. Stambaugh, Leading Change in Gifted Education: The Festschrift of Dr. Joyce Van Tassel Baska. Waco, TX: Prufrock Press. [ Links ]
8. Brody, L. E. (2009b). The Johns Hopkins Talent Search Model for Identifying and Developing Exceptional Mathematical and Verbal Abilities. In L. V. Shavinina, (Ed), International Handbook on Giftedness. New York: Springer. [ Links ]
9. Brody, L. E. & Stanley, J. C. (2005) Youths who Reason Exceptionally well Mathematically and/or Verbally: Using the MVT: D4 Model to Develop their Talents. In R. J. Sternberg & J. E. Davidson (Eds.), Conceptions of Giftedness (2nd ed.). New York: Cambridge University Press. [ Links ]
10. Brody, L. E.; Stanley, J. C.; Barnett, L. B.; Gilheany, S.; Tourón, J. & Pyryt, M. C. (2001). Expanding the Johns Hopkins Talent Search Model Internationally. Gifted and Talent International, 16(2), 94-107. [ Links ]
11. CTY (1995). Academic Acceleration: Knowingyour Options. Baltimore, Md: CTY Publications and Resources, The Johns Hopkins University Press. [ Links ]
12. Duckworth, A. L. & Gross, J. J. (2014). Self-control and Grit: Related but Separable Determinants of Success. Current Directions in Psychological Science, 23(5), 319-325. [ Links ]
13. Dweck, C. (2008). Mindset: the New Psychology of Success. New York: Ballantione cop. [ Links ]
14. Ericsson, K. A. (2007). Deliberate Practice and the Modifiability of Body and Mind: Toward a Science of the Structure and Acquisition of Expert and Elite Performance. International Journal of Sport Psychology, 38, 4-34. [ Links ]
15. Gagné, F. (2009). Building Gifts into Talents: Detailed Overview of the DMGT 2.0. In B. MacFarlane, & T. Stambaugh, (Eds.), Leading Change in Gifted Education: The Festschrift of Dr. Joyce Van Tassel-Baska. Waco, TX: Prufrock Press. [ Links ]
16. Gagné, F. (2015). From Genes to Talent: the DMGT/CMTD Perspective. Revista de Educación, 368, pp. 12-37. [ Links ]
17. Goldstein, D., Stocking, V. B. & Godfrey, J. J. (1999). What We've Learned from Talent Search Research. In N. Colangelo & S. G. Assouline. Talent Development III Proceedings from the 1995 Henry B. and Jocelyn Wallace National Research Symposium on Talent Development. Scottsdale, Arizona: Gifted Psychology Press. [ Links ]
18. Jackson, N. E. & Butterfield, E. C. (1986). A Conception of Giftedness Designed to Promote Research. In R. J. Sternberg & J. E. Davidson (Eds.), Conceptions of Gftedness. Cambridge: Cambridge University Press. [ Links ]
19. Keating, D. P. (1974). The Study of Mathematically Precocious Youth. En J. C. Stanley; D. P. Keating, & L. H. Fox, (Eds.) Mathematical Talent. Discovery, Description, and Development. Baltimore: The Johns Hopkins University Press. [ Links ]
20. Keating, D. P. (Ed.) (1976). Intellectual Talent. Research and Development. Baltimore: The Johns Hopkins University Press. [ Links ]
21. Keating, D. P. & Schaefer, R. A. (1975). Ability and Sex Differences in the Acquisition of Formal Operations. Developmental Psychology, 11(4), 531-532. [ Links ]
22. Keating, D. P. & Stanley, J. C. (1972). Extreme Measures for the Exceptionally Gifted in Mathematics and Science. Educational Researcher, 1, 3-7. [ Links ]
23. Lubinski, D. & Benbow, C. P. (2006). Study of Mathematically Precocious Youth After 35 Years. Uncovering Antecedents for the Development of Math-Science Expertise. Perspectives on Psychological Science, 1(4), 316-345. [ Links ]
24. Lubinski, D., Benbow, C. P. & Sanders, C. E. (1993). Reconceptualising Gender Differences in Achievement among the Gifted. In K. A. Heller; F. J. Monks, & A. H. Passow (Eds.), International Handbook of Research and Development of Giftedness and Talent. London: Pergamon Press. [ Links ]
25. Lubinski, D., Benbow, C.P., & Kell, H. J. (2014). Life Paths and Accomplishments of Mathematically Precocious Males and Females Four Decades Later. Psychological Science, 25, 2217-2232. [ Links ]
26. OCDE (2005). La definición y selección de competencias clave. Resumen ejecutivo (Definition and selection of key competences. Executive summary). In http://www.OECD.org/edu/statistics/deseco. Retrieved January the 30th, 2015. [ Links ]
27. Olszewski-Kubilius, P. (1998). Talent Search: Purposes, Rationale, and Role in Gifted Education. Journal of Secondary Gifted Education, 9(3), 106-113. [ Links ]
28. Olzewski-Kubilius, P.; Subotnik, R. & Worrell, F. (2015). Rethinking Giftedness: A Developmental Approach. Revista de Educación, 368, 245-267. [ Links ]
29. Pfeiffer, S. (2015). Tripartite Model of Giftedness and Best Practices in Gifted Assessment. Revista de Educación, 368, 156-182. [ Links ]
30. Pressey, S. L. (1949). Educational Acceleration: Appraisal and Basic Problems. Columbus, Ohio: The Ohio State University Press. [ Links ]
31. Renzulli, J. & Geasser, A. (2015). A Multi Criteria System for the Identification of High Achieving and Creative/Productive Giftedness. Revista de Educación, 368, 92-121. [ Links ]
32. Reyero, M. & Tourón, J. (2003). El Desarrollo del Talento. La aceleración como estrategia educativa. (Talent Development. Acceleration as Educational Strategy). A Coruña: Netbiblo, 416 pp. [ Links ]
33. Stanley, J. C. (1974). Intellectual Precocity. in J. C. Stanley; D. P. Keating & L. H. Fox, (Eds.), Mathematical Talent. Discovery, Description, and Development. Baltimore: The Johns Hopkins University Press. [ Links ]
34. Stanley, J. C. (1977). Introduction. In J. C. Stanley, W. C. George & C. H. Solano, (Eds.), The Gifted and the Creative. A Fifty Year Perspective 1925-1975. Baltimore: The Johns Hopkins University Press. [ Links ]
35. Stanley, J. C. (1989). Guiding Gifted Students in Their Academic Planning. In J. Van Tassel-Baska, & P. Olszewski-Kubilius, (Eds.). Patterns of Influence in Gifted Learners: the Home, the Self and the School. New York: Teachers College Press. [ Links ]
36. Stanley, J. C. (1990a). Finding and Young People with Exceptional Mathematical Reasoning Ability. In M. AT. Howe (Ed.), Encouraging the Development of Exceptional Skills and Talents. Leicester, England: British Psychological Society. [ Links ]
37. Stanley, J. C. (1990b). Leta Hollingworth´s Contributions to Above-Level Testing of the Gifted. Roeper Review, 12(3), 166-171. [ Links ]
38. Stanley, J. C. (1991). SMPY's Identification, Motivation, and Educational Facilitation Model. In R. E. Clasen, Educating Able Learners: Book of Readings. Madison, WI: Madison Education Extension Programs, University of Wisconsin-Madison. [ Links ]
39. Stanley, J. C. (1996). In the Beginning: The Study of Mathematically Precocious Youth. In C. P. Benbow, & D. Lubinski, Intellectual Talent. Psychometric and Social Issues. Baltimore: The Johns Hopkins University Press. [ Links ]
40. Stanley, J. C. (2001). Helping Students to Learn what They Don't Already Know. Proceedings from the 1998 Henry B. and Jocelyn Wallace National Research Symposium on Talent Development. Scottsdale: Great Potential Press. [ Links ]
41. Stanley, J. C. (2005). A Quiet Revolution: Finding Boys and Girls who Reason Exceptionally well Intellectually and Helping them get the Supplemental Educational Opportunities they Need. High Ability Studies, 16(1), 5-14. [ Links ]
42. Stanley, J. C. & Benbow, C. P. (1983). Extremely Young College Graduates: Evidence of Their Success. College and University, 58, 361-371. [ Links ]
43. Stanley, J. C. & Benbow, C. P. (1986). Youths Who Reason Exceptionally Well Mathematically. In R. J. Sternberg, & J. E. Davidson, (Eds.), Conceptions of Giftedness. New York: Cambridge University Press. [ Links ]
44. Stanley, J. C. & Brandt, R. S. (1981). On Mathematically Talented Youth: A Conversation with Julian Stanley. Educational Leadership, 39(1), 101-106. [ Links ]
45. Stanley, J. C. & George, W. C. (1978). Now We Are Six: The Ever-Expanding SMPY. Gifted Child Today, 1, 9-11. [ Links ]
46. Tourón, J. (2001). School and College Ability Test (SCAT) Validation in Spain: Overview of the Process and some Results. Gifted and Talented International, 16(2), 104-107. [ Links ]
47. Tourón, J. (2005) (Ed.). The Center for Talented Youth Model. Special Issue. High Ability Studies, 16 (1), 1-158. [ Links ]
48. Tourón, J. (2009). El establecimiento de estándares de rendimiento en los sistemas educativos (Achievement Standards implementation in Education Systems). Estudios sobre Educación, 16, 127-146. [ Links ]
49. Tourón, J. & Reyero, M. (2002). The Implementation of the Talent Search Concept in Spain.NATO Science, Volume No. 977925, 63-76. [ Links ]
50. Tourón, J. & Santiago, R. (2013). Atención a la diversidad y desarrollo del talento en el aula. El modelo DT-PI y las tecnologías en la implantación de la flexibilidad curricular y el aprendizaje al propio ritmo (Attention to Diversity and Talent Development in the Classroom. The DT-PI Model in the Implementation of Curricular Flexibility and Self-paced Learning). Revista Española de Pedagogía, año LXXI, no 256, 441-459. [ Links ]
51. Tourón, J. & Santiago, R. (2015). The Flipped Learning Model and Talent Development in Schools. Revista de Educación, 368, pp. 176-208. [ Links ]
52. Tourón, J. & Tourón, M. (2006). La identificación del talento verbal y matemático de los jóvenes más capaces: el modelo de CTY España (Identification of Verbal and Math Talent of the more able: CTY Spain model). I Simposio Internacional sobre Altas capacidades. Consejería de educación. Las Palmas de Gran Canaria. Noviembre. [ Links ]
53. Tourón, J. & Tourón, M. (2011). The Center for Talented Youth Identification Model: A Review of the Literature. Talent Development & Excellence, 3(2), 187-202. [ Links ]
54. Tourón, J. Tourón, M. & Silvero, M. (2005). The Center for Talented Youth Spain: an Initiative to Serve Highly Able Students. High Ability Studies, 16(1), 121-135. [ Links ]
55. Tourón, J.; Peralta, F. & Repáraz, Ch. (1998). La superdotación intelectual. Modelos, identificación y estrategias educativas (Intellectual Giftedness. Models, Identification and Educational Strategies). Pamplona: EUNSA. [ Links ]
![]() Correspondence:
Correspondence:
Javier Tourón.
Universidad Internacional de la Rioja.
Gran Vía Rey Juan Carlos I, 41.
26002, Logroño, La Rioja (Spain).
E-mail: Tavier.Touron@unir.net
Article received: 30-09-2015
Revised: 11-05-2016
Accepted: 26-05-2016