Mi SciELO
Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO -
 Accesos
Accesos
Links relacionados
-
 Citado por Google
Citado por Google -
 Similares en
SciELO
Similares en
SciELO -
 Similares en Google
Similares en Google
Compartir
Revista de Osteoporosis y Metabolismo Mineral
versión On-line ISSN 2173-2345versión impresa ISSN 1889-836X
Rev Osteoporos Metab Miner vol.8 no.2 Madrid abr./jun. 2016
Analysis of mechanical behavior variation in the proximal femur using X-FEM (Extended Finite Element Method)
Análisis de la variación del comportamiento mecánico de la extremidad proximal del fémur mediante el método XFEM (eXtended Finite Element Method)
Marco M.1, Giner E.2, Larraínzar R.3, Caeiro J.R.4 and Miguélez H.1
1 Departamento de Ingeniería Mecánica - Universidad Carlos III - Madrid (España)
2 Universidad Politécnica de Valencia - Centro de Investigación en Ingeniería Mecánica CIIM - Departamento de Mecánica y de Materiales - Valencia (España)
3 Hospital Universitario Infanta Leonor - Servicio Cirugía Ortopédica y Traumatología - Madrid (España)
4 Complejo Hospitalario Universitario de Santiago de Compostela - Servicio de Cirugía Ortopédica y Traumatología - A Coruña (España)
Work submitted as FEIOMM benefit the grant received to attend the 35th Congress of the ASBMR (Baltimore, 2013).
SUMMARY
Introduction: For years, the human femur has been extensively studied experimentally with in vitro analysis. Nowadays, with computer advances, it can also be analyzed numerically. Some authors report the usefulness of finite method in predicting the mechanical behavior of this bone. There are many possibilities using the synergy between the method finite element and experimental trials. In this paper, for example, we study how they affect different osteoporotic simulations involving femur fracture loads.
The aim of this study is to predict hip fracture, both the load to which this occurs as the propagation of the crack in the bone. By applying the finite element method to the field of bio-mechanics, simulation can be carried out to show the behavior under different bone load conditions.
Material and methods: Using DICOM images, CT scan of the proximal end of the right femur of a male has been obtained bone geometry. By a computer program they have been generated dependent mechanical properties of the BMD each voxel, and then used a finite code to apply different load configurations and study values bone fracture elements. The numerical model has been validated in the literature.
Results: Load breaking in lateral fall configuration is approximately half the load in the case of the normal position, which agrees with different experimental studies published.
In addition, we have studied various load conditions in everyday situations, where it was observed that the load fracture is minimal in mono-podal position. Osteoporotic conditions have also been simulated which confirmed that the load fracture has been reduced by decreasing mechanical properties.
Conclusions: By using the finite element method in conjunction with DICOM medical imaging, it is possible to study the biomechanics of the hip and obtain an estimate of bone failure. In addition, different load configurations can be applied and vary the mechanical properties of bone to simulate the mechanical behavior of low osteoporotic conditions.
Key words: femur, hip fracture, CT scanner, finite elements.
RESUMEN
Introducción: El fémur humano ha sido ampliamente estudiado desde hace muchos años de manera experimental con análisis in vitro, y ahora, gracias a los avances de la informática, también se puede analizar de manera numérica. Algunos autores han demostrado la capacidad del método de los elementos finitos para predecir el comportamiento mecánico de este hueso, pero todavía son muchas las posibilidades recurriendo a la sinergia entre el método de los elementos finitos y ensayos experimentales. En este trabajo, por ejemplo, se estudia cómo afectan distintas simulaciones de osteoporosis a las cargas de fractura del fémur.
El objetivo de este estudio es predecir la fractura de cadera, tanto la carga a la que se produce ésta como la propagación de la fisura sobre el hueso. Aplicando el método de los elementos finitos al campo de la biomecánica se puede realizar una simulación que muestre el comportamiento del hueso bajo diferentes condiciones de carga.
Material y métodos: A partir de imágenes DICOM de tomografía computarizada de la extremidad proximal del fémur derecha de un varón se ha obtenido la geometría del hueso. Mediante un programa informático se han generado las propiedades mecánicas dependientes de la densidad mineral ósea de cada vóxel, y posteriormente se ha utilizado un código de elementos finitos para aplicar diferentes configuraciones de carga y estudiar los valores de fractura del hueso. El modelo numérico ha sido validado a través de un artículo de la literatura científica.
Resultados: La carga de fractura en configuración de caída lateral es aproximadamente la mitad que la carga en el caso de la posición normal, lo cual concuerda con diferentes estudios experimentales presentes en la literatura científica. Además se han estudiado diferentes condiciones de carga en situaciones cotidianas, en las que se ha observado que la carga de fractura es mínima en la posición monopodal. También se han simulado condiciones de osteoporosis en las que se ha comprobado cómo desciende la carga de fractura al disminuir las propiedades mecánicas óseas.
Conclusiones: Mediante el método de los elementos finitos en conjunto con una imagen médica DICOM es posible el estudio de la biomecánica de la cadera y obtener una estimación del fallo del hueso. Además se pueden aplicar diferentes configuraciones de carga y variar las propiedades mecánicas del hueso para simular el comportamiento mecánico de éste bajo condiciones osteoporóticas.
Palabras clave: fémur, fractura de cadera, escáner CT, elementos finitos.
Introduction
According to recent information sources available in Spain [1], a total of 487,973 cases of hip fracture were reported between 1997 and 2008. There is a female predominance of 3 to 1 [2] and the incidence increases with age (in 1997, it was 78.07 years, while in 2009 it increased to 80.46 years). Proximal femoral fractures (PFF) imply high health costs due to the long-term average stay (in 1997, the average was 16.05 days, while in 2008 the figure fell to 13.34 days) and the direct expenditure this entails. In 2008, the overall cost of hospitalizations in Spain's National Health System was 395.7 million euros. This was a 131% increase compared with 1997. Individually, the cost per patient rose from 4,909 euros in 1997 to 8,365 euros per patient in 2008. It should be noted that the fracture also carries an acutely high mortality rate of hospital care (4.71 to 5.85%) as well as a year later (25-33%) [3].
In this context, the need for predictive methods of fracture arises, both at individual and population-wide levels. The prediction of femoral fracture is a challenge in the world of biomechanics, with both doctors and engineers focusing on the study of crack propagation in human bones. For years there have been experimental analyses of human femurs from donors. Today, with technological advances, computers are more sophisticated and able to enhance this analysis, reducing experimental study costs. At the same time, we can better understand the processes of how cracking and fractures appear. With a finite element model, scientists can study the mechanical behavior of the femur under conditions of specific load and therefore assess the normal biomechanics of the hip and pathophysiological process fracture with a correlation of about 90% [4] despite a great variability in the choice of the mechanical properties that apply to the different numerical models studied to date [5,6].
The main objective of our study is to develop a PFF model using finite elements to analyze the different configurations required under normal and pathological load. As secondary objectives, we want to evaluate the morphology and minimum load configuration from which the dependent load conditions fracture is initiated and analyzed numerically how it affects osteoporosis breaking load bone, due to the reduced mechanical properties arising as a result of bone mass loss [7].
Material and method
Generation of model of finite elements
From computed tomography (CT) DICOM images of the right PFF of a young adult male without a known hip condition in the studied side, the macro-structure geometry of the bone was obtained. Medical images were obtained with a radiation dose and standard clinical exposure time enabling a resolution of 0.3 mm in the transverse plane and 0.7 mm in the longitudinal direction.
The femoral geometry was generated by image processing software and ScanIP finite element modeling (Simpleware, Exeter, UK). This allows us to select the range of Hounsfield unit (HU) scale necessary for proper display of CT images, applying volume/surface filters and generating an identical geometry to the specimen resulting in a numerical model with mechanical properties dependent on bone mineral density (BMD) supported in the following equations [8-11]:
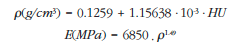
Through these expressions, a relationship is established between the HU of each voxel, its density and Young's modulus (E). In this case, the model has 14 different materials in order to reproduce the maximum heterogeneity of real bone. Using these equations, the bone will present isotropic behavior, which does not really reflect reality, but, as has been shown in other studies, we can simulate the behavior of the femur globally through these expressions.
Loading conditions of the finite element model
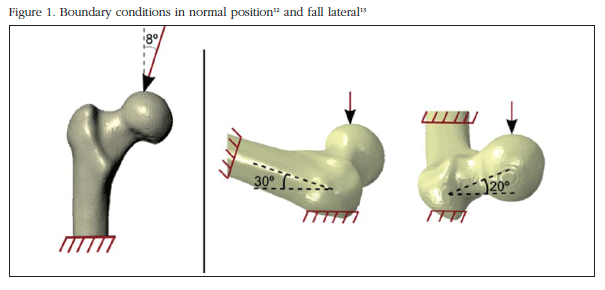
The finite element analysis of the PFF generated is carried out using the Abaqus/Standard 6.12 program. (Dassault Systems, Providence, Rhode Island). The discretized mesh volume is formed by 198,764 second-order tetrahedral elements (C3D10 in Abaqus). The PFF area has a finer mesh of 2 mm while the rest of the model elements are 3 mm in size. The nodes of the bottom of the PFF are embedded (prevented from displacement in any direction). As shown in figure 1, it has been considered normal loading position that it assumes that the load vector has an angle of 8o adducted with the longitudinal axis of the hip in transversal plane [12]. lateral fall is considered one that is a load vector with a rotation of 20o in ante-version and 30o to the longitudinal axis as pivot point [13].
Validation of the numerical model
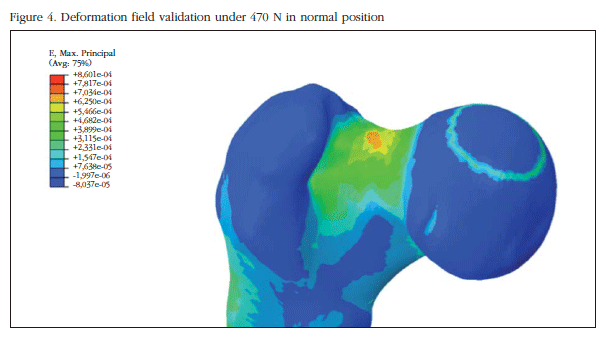
Results were validated by comparing values obtained in two different configurations: normal and lateral position with those obtained in similar conditions in experimental work in a human corpse [8]. To this end we assume that the initial bone break occurs when a critical deformation of 0.0061 [14] is reached. The magnitude of the validation load is 470 N (75% of the individual's weight).
Loading boundary conditions and patterns of fracture in standing position and lateral fall
To analyze femoral fracture patterns in standing position and lateral fall, the Extended Finite Element Method (XFEM) implemented in Abaqus was used. With this method, the start of a crack is determined in the maximum deformation area. Then this field is spread depending on the stresses and strains surrounding it. The parameters of fracture toughness (density dependent) and the critical energy for propagation in different modes are obtained in the literature, according to the following formulas [15,16]:
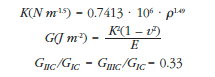
Boundary conditions and breaking loads in normal locomotor activities
For analysis of stresses in various daily life activities, the fixed shaft is considered. Calculations are carried out according to the different angles at which the load is applied in the PFF, as reported by Bergmann [17]. In all, 9 different configurations are analyzed: monopodal support, climbing stairs, walking slowly down stairs, walking fast, regular walking, standing, bending knees and sitting.
Boundary conditions and loads of simulated fracture in osteoporosis
For stress analysis under simulated osteoporosis, the BMD model has been decreased and therefore a decrease of Young's modulus and overall femoral stiffness. BMD variation is carried out in different areas: in the PFF generally at the femoral neck, trochanteric area in the upper area of the shaft and in the middle of the shaft. The loading conditions are performed in the normal position of the PFF analyzing how this affects a loss of tissue stiffness, as that could be caused by osteoporosis, the breaking load of the PFF. Figure 2 the areas where BMD was varied and the boundary conditions corresponding to the loading position under study.
Results
Finite element model of the PFF
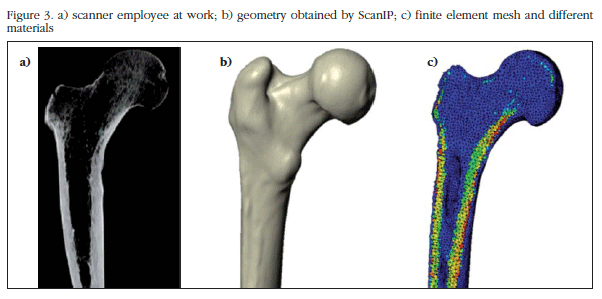
The finite element model of the PFF derived from DICOM medical images is shown in figure 3. The model was generated by ScanIP software; in figure 3b the femoral surface was observed, and in figure 3c, the heterogeneity model.
Validation of the numerical model
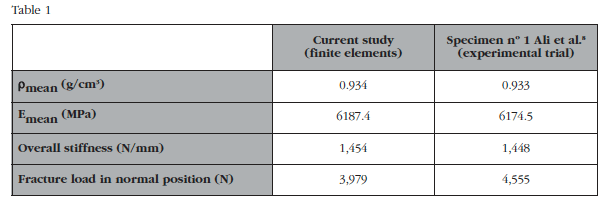
Table 1 shows the general properties assigned to the numerical model and the results in stiffness and breaking loads between the developed model and the comparator chosen. The mechanical properties assigned are virtually identical to those calculated in the experimental study that supports this article[8]. It is noted that both overall rigidity and fracture load in normal position are similar to the experimental test, therefore the numerical model may be considered validated. In figure 4 the strain field analysis obtained in this validation is shown.
Standing fracture patterns and lateral fall
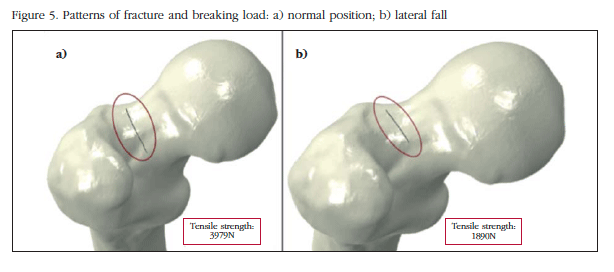
Figure 5 shows the pattern of fracture start under the conditions described. It can be seen how the breaking load in lateral fall configuration is lower than in the normal position (around 50% lower, of 3,979 N to 1,890 N). In both cases the crack starts at the top of the femoral neck, although in the case of lateral crash onset is more posterolateral neck.
Boundary conditions and breaking loads in normal locomotor activities
Figure 6 shows the breaking load for each of the analyzed configurations discussed above. The load at which the critical strain is reached and at which fracture occurs is ordered from lowest to highest for easy viewing of data.
This clearly shows how the most critical configuration is corresponding to the lateral drop previously studied, followed by the normal position. The other positions have a higher breaking load, though not much variation as the lateral fall. In the configuration of the lateral fall, load value decreases considerably, assuming at least half load in the remaining cases.
Boundary conditions and simulated loading fracture in osteoporosis
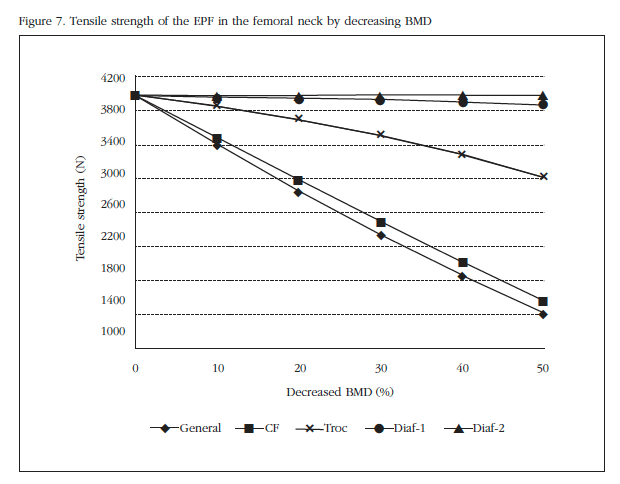
Under the conditions described osteoporosis simulated by a percentile decrease of mechanical properties in different areas of the PFF weakening that occurs in bone structure by reducing the stiffness of this because of osteoporosis objective. Figure 7 shows the breaking load according to the decrease in BMD (has decreased to 50% of initial density).
This indicates how the most critical decline is related to the changed properties in the overall PFF, but also shows how the decreased BMD only in the neck area, the variation that the breaking load suffers is virtually identical to the general case. The trochanter area also proves be critical, although not as much as those already mentioned, while in areas of the diaphysis the reduction practically does not affect the breaking load, due to its distance from the neck area and the analyzed boundary conditions in particular. It shows how the BMD decrease is a great variation in the fracture load, reducing it by more than half for a 50% BMD decrease.
Discussion
In this study, a complete finite element model has been developed to predict the PFF failure and simulate the fracture that occurs depending on load conditions. The breaking load at different positions was also obtained for everyday life. These were compared to those of the lateral fall along with the effect of decreasing BMD and load necessary for breaking. Thus we have developed a computational model that allows experimental study of the proximal femur.
The method of developing the geometric structure from medical imaging has been used in other published studies [8-11]. Choosing a young adult patient is justified in order to obtain a proper numerical value transfer of bone density that represents a proximal femur under physiological conditions, and suitable from the biomechanical point of view. The choice of a patient of advanced age would seem a more clinically realistic hip fracture problem, but would not represent the standard physiological pattern of the proximal femur.
Having a geometric numerical model allows for changes in load conditions, intensity and vector, boundary conditions and mechanical strength properties. We believe that the 470 Newton load chosen for analyzing the different configurations is appropriate because it corresponds to the estimated value of a young adult who is the starting point of the model described. The vector load application in the standing position is admitted to several experimental studies, as is the vector application in lateral fall.
As in any numerical model, proper validation is required to ensure that that results achieved are close to reality. We believe that the proposed validation compared to experimental test results made by other authors, is appropriate because these mechanical stresses involve different human femurs and study their overall stiffness and breaking load in the normal position. The results Analysis of the results obtained it can be concluded that the model reasonably reproduces reality, and other results can be obtained by modifying boundary conditions, properties, etc. In addition, the maximum deformation occurs in the upper femoral neck. Several other studies reached this same conclusion [8,18].
The model shows that in both standing and lateral fall, the crack starts in the upper region of the femoral neck. As described in the literature, this actually shows that the bone better supports compression loads than those of traction. In the femoral neck in standing position this happens. In the lower area, compressive loads occur while in the upper region, they are related to traction. We believe that the similarity between the mathematical prediction and the one expected reinforces the validity of the methodology used. The femoral neck is not circular but oval, with the largest cortical thickness at the bottom rather than at the top. In our case, being a young adult, there is a big difference in the starting point between the normal position and lateral fall, because the difference between cortical thickness between the upper and lower areas is not very high. Probably if we had used images for an elderly patient, the starting fracture point in lateral fall would have been even more posterolateral in the femoral neck.
The breaking loads for normal position and lateral fall within a usual range have been demonstrated experimentally in other studies [4,8,19,20]. The values for the breaking load fall on the side are 50% lower than in the normal position, consistent with experimental results [4,8]. Failure criteria considered are able to detect the area where the numerical model fracture starts. We also consider the presence of the crack and predict its spread by XFEM, although it is true that a complete femoral fracture due to problems of convergence in the solution is not achieved.
The breaking loads in the femur for different positions of everyday life whose load angles were obtained in the work of Bergmann et al. [17] had not been studied so far, and is a new source of information and study.
The results show how the femur is optimized for loads under physiological conditions; morphology and anisotropy make major stresses and strains converge towards alignment, allowing it greater load support. Instead, under an abnormal load, as is the case of a lateral falls, the charges are not aligned with the direction of the femoral support [21]. So we see how the fracture load for actions of everyday life is much higher than for non-physiological conditions, such as a lateral falls as there is logical to think that the femur is adapted to the loads of daily life.
From the macroscopic point of view, the 3 factors that most affected PFF strength are its geometry, bone mineral density and traumatic load fall. It is obvious that reducing the BMD of the model entails a lower breaking load to produce fracture, as indeed happens in reality. The ability to reduce the density globally or in specific areas opens a new line of research that may correlate the experimental work in which it is known that the trabecular bone provides much less bone strength in the PFF than the cortical bone.
Almost most clinical hip fractures occur in the trochanter area or neck. Interestingly, these two areas are the most sensitive to the simulated decrease in zonal BMD values. This means that the breaking loads are significantly lower and therefore there is a high clinical prevalence. These findings reinforce the validity of the model developed in predicting fracture.
We are aware of the limitations of the work; the model represents the analysis of a single patient and their particular conditions. This is especially interesting in individual predictions but we cannot infer age correspondence with other groups, morphotypes or gender. Furthermore, the model does not provide any lateral fall of existing dampers in reality, such as static because soft tissue or due to dynamic osteo-tendinous reflection. Undoubtedly, adding these dampering factors to the numerical geometric pattern would correspond to a higher correlation with reality, but their absence does not invalidate the findings.
We believe that the proposed model represents the first step of our research group and has allowed us to define the procedure for the experimental and numerical study of the proximal end of the femur. In the future human femurs will be studied under normal conditions and compared to other senile groups, to analyze the influence of aging bone, both in the breaking load and its corresponding pattern.
Conclusions
• Using the finite element method and a computer program able to get the geometry and distribution of mechanical properties using a CT scan, we can predict failure with valid PFF results in different load configurations.
• By studying different loading configurations, we see how the most critical load corresponds to the lateral fall (50% lower than in the normal position). Other positions of daily life have a load greater than previous fracture. This is because the geometry of the femur has evolved to support common loads (normal position and the remaining positions of daily living) instead of lateral falls.
• By simulating conditions in different areas of osteoporosis, we observed how uniformly decreasing properties are most critical in terms of the breaking load. The next most critical area is that of the femoral neck, which shows that it is vital in the PFF structure. The area of the shaft has been the least influential in this study.
Competing interests: The authors declare no conflict of interest in connection with this work.
![]() Correspondence:
Correspondence:
Miguel Marco Esteban
Universidad Carlos III de Madrid
Departamento de Ingeniería Mecánica
Avda. de la Universidad, 30
28911 Leganés - Madrid (España)
e-mail: mimarcoe@ing.uc3m.es
Date of receipt: 25/11/2015
Date of acceptance: 15/03/2016
Bibliography
1. Instituto de Información Sanitaria. Estadísticas comentadas: La Atención a la Fractura de Cadera en los Hospitales del SNS (Publicación en Internet). Madrid: Ministerio de Sanidad y Política Social; 2010. Disponible en: httsps.es/estadEstudios/estadisticas/cmbdhome.htm. [ Links ]
2. McCreadie BR, Morris MD, Chen T-C, Sudhaker Rao D, Finney WF, Widjaja E. Bone tissue compositional differences in women with and without osteoporotic fracture. Bone. 2006;39:1190-5. [ Links ]
3. Carpintero P, Caeiro JR, Carpintero R, Morales A, Silva S, Mesa M. Complications of hip fractures: A review. World J Orthop. 2014;5(4):402-11. [ Links ]
4. Schileo E, Balistreri L, Grassi L, Cristofolini L, Taddei F. To what extent can linear finite element models of human femora predict failure under stance and fall loading configurations? J Biomech. 2014;47:3531-8. [ Links ]
5. Marco M, Rodríguez-Millán M, Santiuste C, Giner E, Miguélez H. A review on recent advances in numerical modelling of bone cutting. J Mech Behav Biomed Mater. 2015;44:179-201. [ Links ]
6. Giner E, Arango C, Vercher A, Fuenmayor FJ. Numerical modelling of the mechanical behaviour of an osteon with microcracks. J Mech Behav Biomed Mater. 2014;37:109-24. [ Links ]
7. Lubarda VA, Novitskaya EE, McKittrick J, Bodde SG, Chen PY. Elastic properties of cancellous bone in terms of elastic properties of its mineral and protein phases with application to their osteoporotic degradation. Mechanics of Materials. 2012;44:139-50. [ Links ]
8. Ali AA, Cristofolini L, Schileo E, Hu H, Taddei F, Kim RH, Rullkoetter PJ, Laz P. Specimen-specific modeling of hip fracture pattern and repair. J Biomech. 2013;47,536-43. [ Links ]
9. Keyak JH, Falkinstein Y. Comparison of in situ and in vitro CT scan-based finite element model predictions of proximal femoral fracture load. Med Eng Phys. 2003;25:781-7. [ Links ]
10. Schileo E, Taddei F, Cristofolini L, Viceconti M. Subject-specific finite element models implementing a maximum principal strain criterion are able to estimate failure risk and fracture location on human femurs tested in vitro. J Biomech. 2008;41:356-67. [ Links ]
11. Ural A, Bruno P, Zhou B, Shi XT, Guo XE. A new fracture assessment approach coupling HR-pQCT imaging and fracture mechanics-based finite element modeling. J Biomech. 2013;46:1305-11. [ Links ]
12. Cristofolini L, Schileo E, Juszczyk M, Taddei F, Martelli S, Viceconti M. Mechanical testing of bones: the positive synergy of finite-element models and in vitro experiments. Philos Trans R Soc A Math Phys Eng Sci. 2010;368:2725-63. [ Links ]
13. Keyak JH. Relationships between femoral fracture loads for two load configurations. J Biomech. 2000;33:499-502. [ Links ]
14. Morgan EF, Keaveny TM. Dependence of yield strain of human trabecular bone on anatomic site. J Biomech. 2001;34:569-77. [ Links ]
15. Zimmermann E, Launey ME, Barth HD, Ritchie RO. Mixed-mode fracture of human cortical bone. Biomaterials. 2009;30:5877-84. [ Links ]
16. Cook RB, Zioupos P. The fracture toughness of cancellous bone. J Biomech. 2009;42:2054-60. [ Links ]
17. Bergmann G, Deuretzbacher G, Heller M, Graichen F, Rohlmann A, Strauss J, et al. Hip contact forces and gait patterns from routine activities. J Biomech. 2001;34:859-71. [ Links ]
18. Doblaré M, García JM, Gómez MJ. Modelling bone tissue fracture and healing: a review. Eng Fract Mech. 2004;71:1809-40. [ Links ]
19. Juszczyk MM, Cristofolini L, Salva M, Zani L, Schileo S, Viceconti M. Accurate in vitro identification of fracture onset in bones: Failure mechanism of the proximal human femur. J Biomech. 2013;46:158-64. [ Links ]
20. Zani L, Erani P, Grassi L, Taddei F, Cristofolini L. Strain distribution in the proximal Human femur during in vitro simulated sideways fall. J Biomech. 2015;48:2130-43. [ Links ]
21. Cristofolini L, Juszczyk M, Zani L, Viceconti M. For which loading scenarios is the proximal femur optimized? J Biomech. 2012;45(S1):S283. [ Links ]











 texto en
texto en